Задание
Построение графика квадратичной функции \(\displaystyle y=x^2\) на отрезке \(\displaystyle [-1;\, 1] \small .\)
Решение
Заполним таблицу значений квадратичной функции \(\displaystyle \small y=x^2{\small :}\)
| \(\displaystyle \small x\) | \(\displaystyle \small -1\) | \(\displaystyle \small -0{,}8\) | \(\displaystyle \small -0{,}6\) | \(\displaystyle \small -0{,}4\) | \(\displaystyle \small -0{,}3\) | \(\displaystyle \small 0\) | \(\displaystyle \small 0{,}3\) | \(\displaystyle \small 0{,}4\) | \(\displaystyle \small 0{,}6\) | \(\displaystyle \small 0{,}8\) | \(\displaystyle \small 1\) |
| \(\displaystyle \small y=x^2\) | \(\displaystyle \small \small (-1)^2\) | \(\displaystyle \small (-0{,}8)^2\) | \(\displaystyle \small (-0{,}6)^2\) | \(\displaystyle \small (-0{,}4)^2\) | \(\displaystyle \small (-0{,}3)^2\) | \(\displaystyle \small 0\) | \(\displaystyle \small 0{,}3^2\) | \(\displaystyle \small 0{,}4^2\) | \(\displaystyle \small 0{,}6^2\) | \(\displaystyle \small 0{,}8^2\) | \(\displaystyle \small 1^2\) |
Вычисляем значения:
| \(\displaystyle \small x\) | \(\displaystyle \small -1\) | \(\displaystyle \small -0{,}8\) | \(\displaystyle \small -0{,}6\) | \(\displaystyle \small -0{,}4\) | \(\displaystyle \small -0{,}3\) | \(\displaystyle \small 0\) | \(\displaystyle \small 0{,}3\) | \(\displaystyle \small 0{,}4\) | \(\displaystyle \small 0{,}6\) | \(\displaystyle \small 0{,}8\) | \(\displaystyle \small 1\) |
| \(\displaystyle \small y=x^2\) | \(\displaystyle \small 1\) | \(\displaystyle \small 0{,}64\) | \(\displaystyle \small 0{,}36\) | \(\displaystyle \small 0{,}16\) | \(\displaystyle \small 0{,}09\) | \(\displaystyle \small 0\) | \(\displaystyle \small 0{,}09\) | \(\displaystyle \small 0{,}16\) | \(\displaystyle \small 0{,}36\) | \(\displaystyle \small 0{,}64\) | \(\displaystyle \small 1\) |
Построим точки на плоскости:
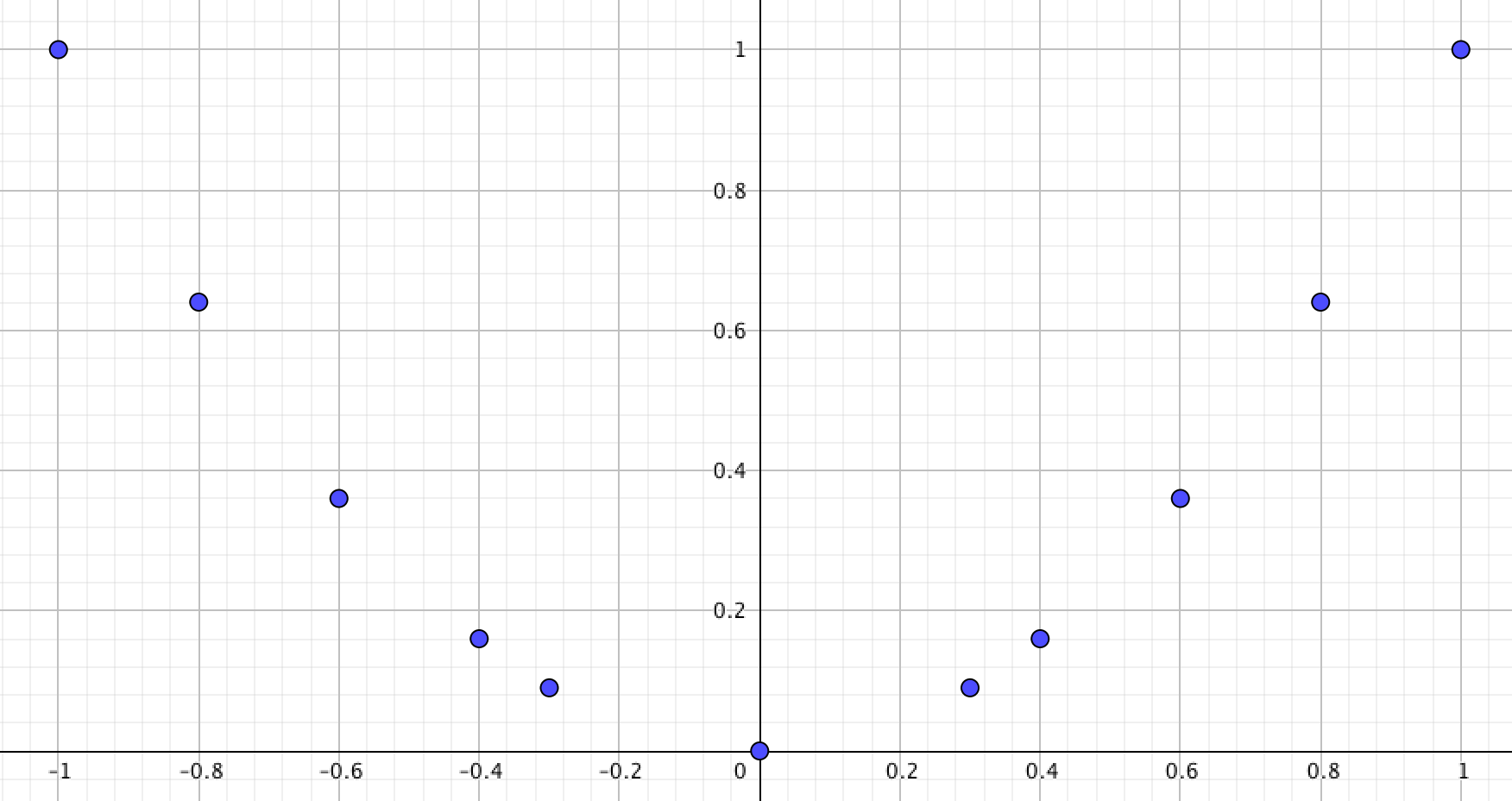
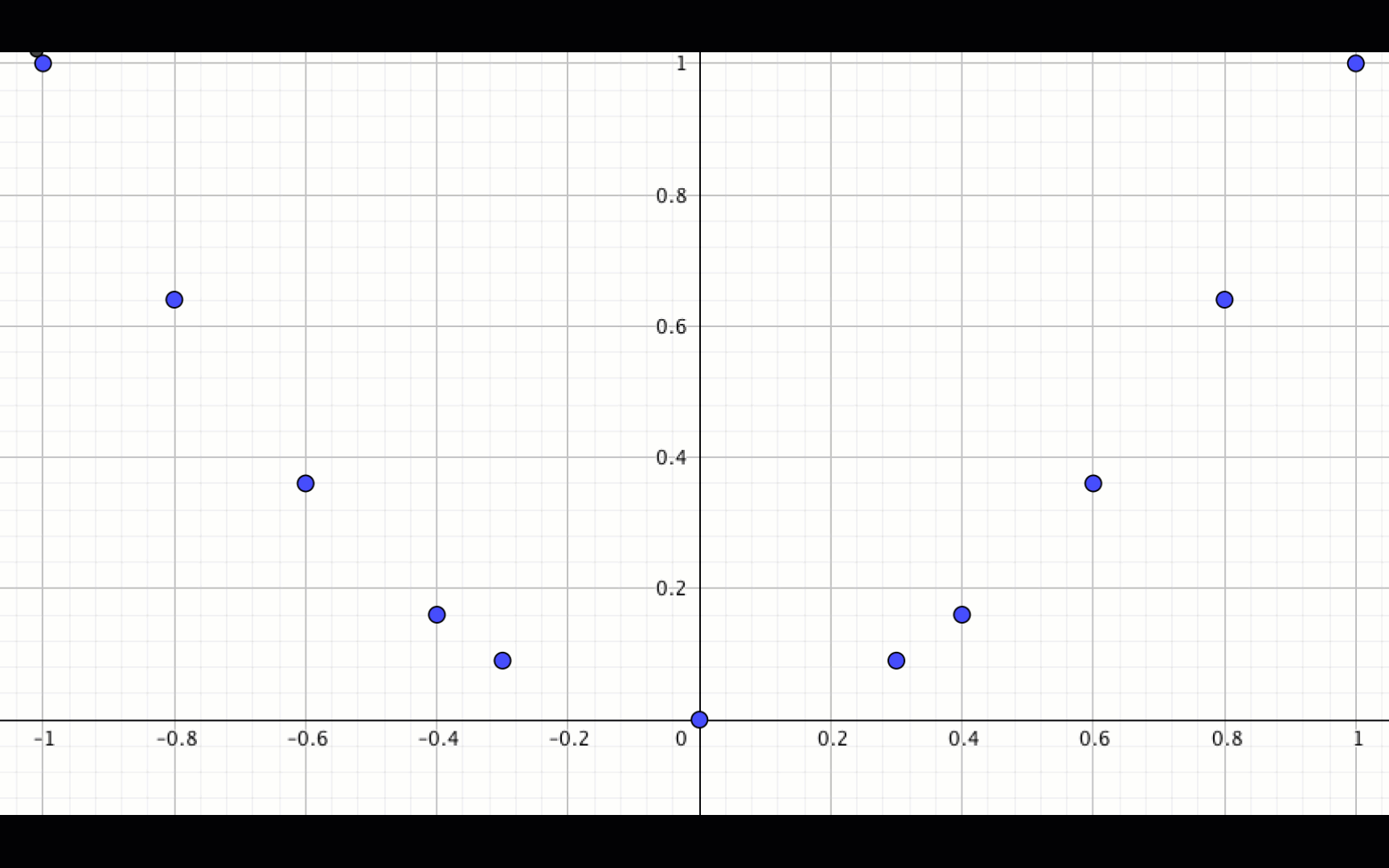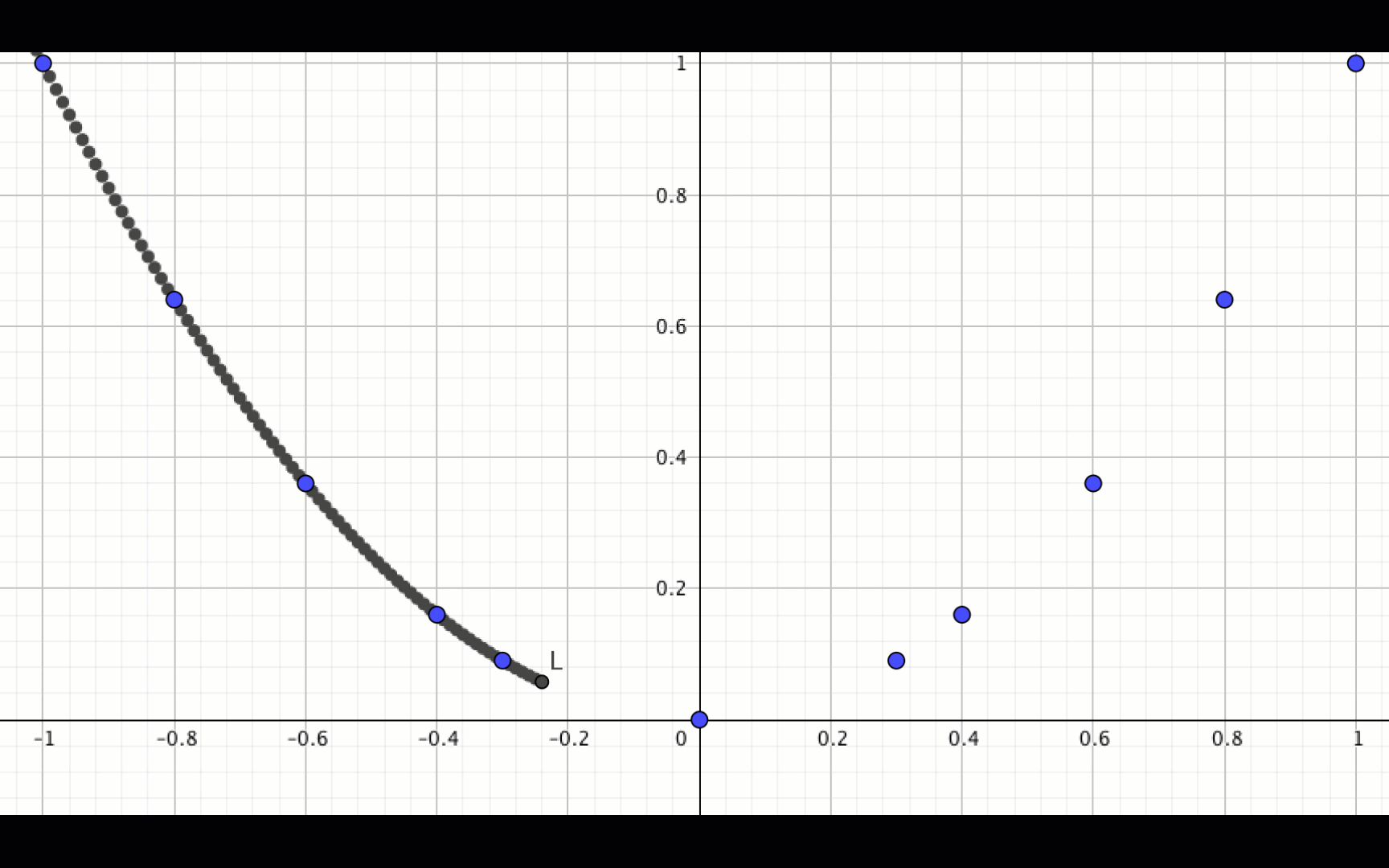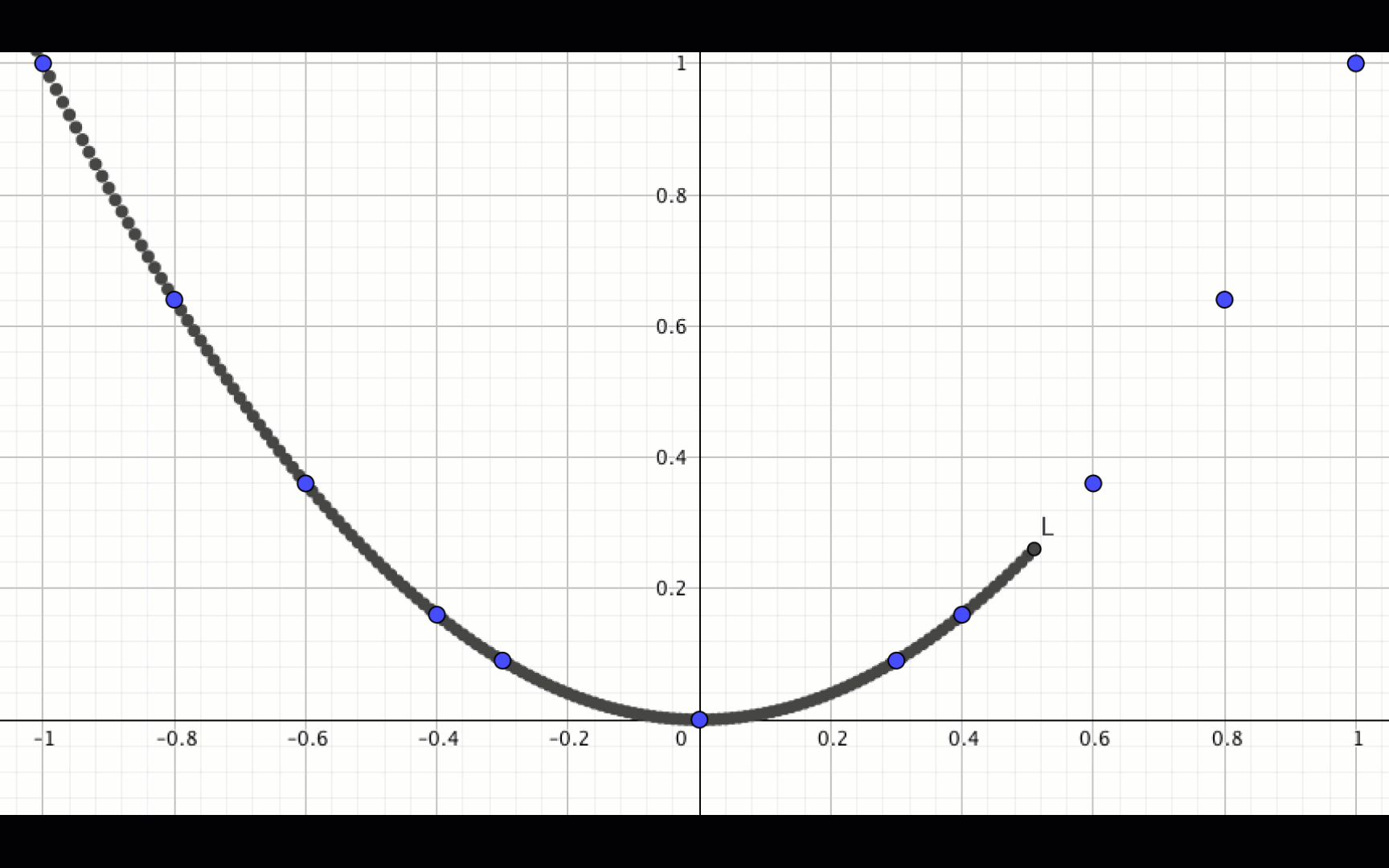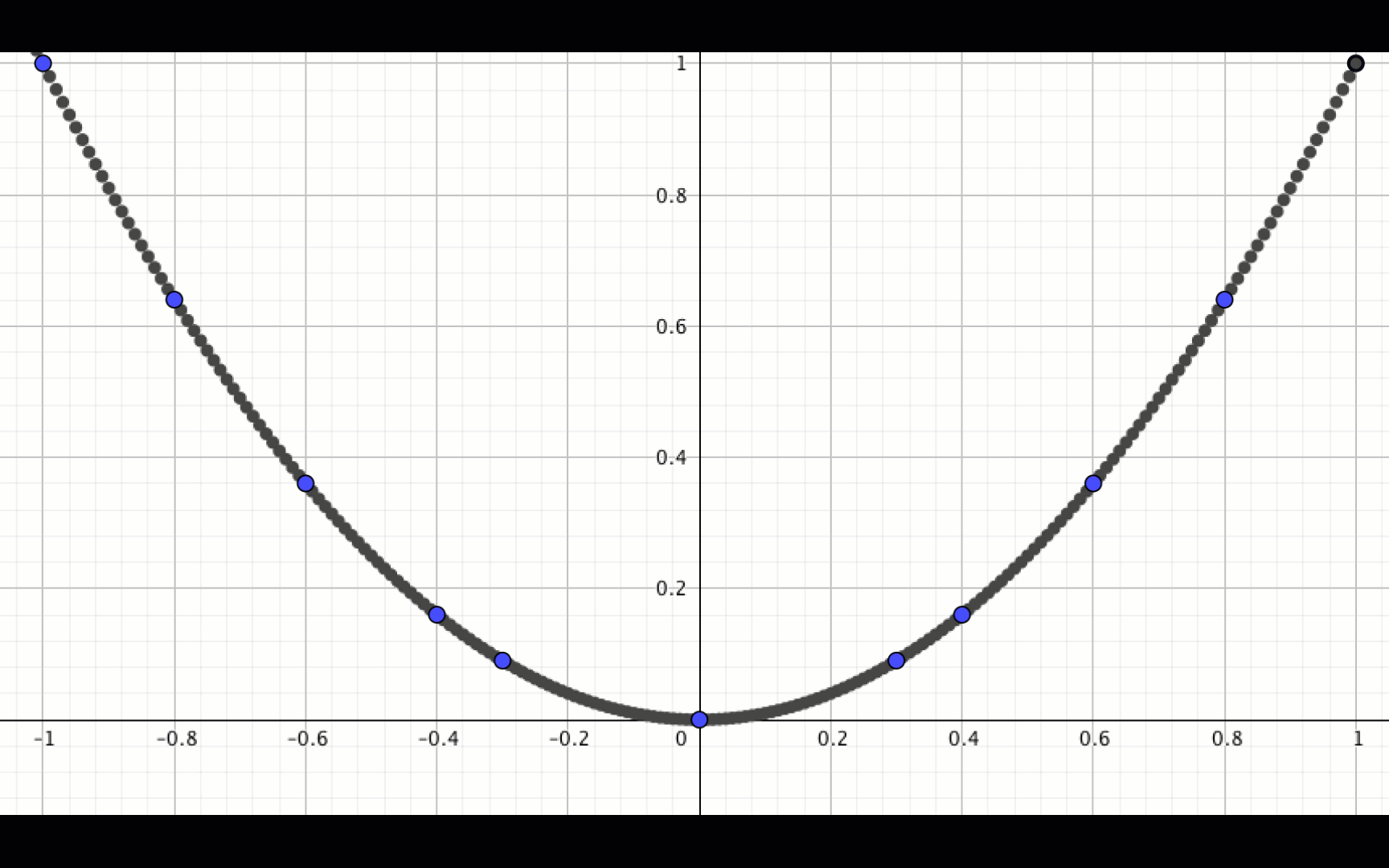
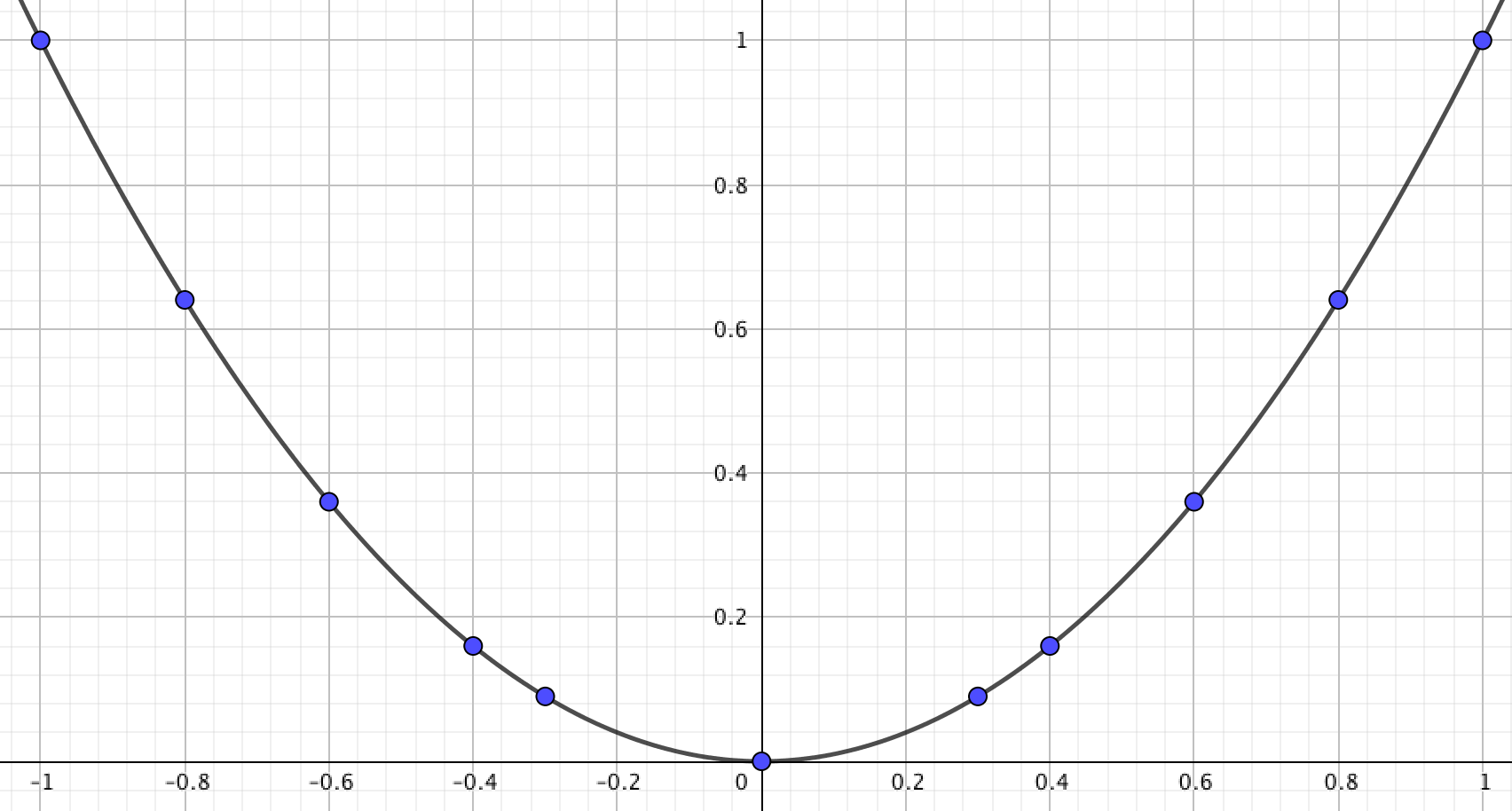
Построим график квадратичной функции \(\displaystyle \small y=x^2\) по полученным точкам, добавляя еще точки, если это необходимо:
Замечание / комментарий
Построение по точкам
Если построить по оси ОХ много точек с координатами от \(\displaystyle \small -1 \) до \(\displaystyle \small 1{\small , } \) то получаем следующую картинку графика: