Үшбұрыштың \(\displaystyle AB \) жағындағы \(\displaystyle D \) нүктесі \(\displaystyle AD=AC {\small}\) таңдалады. Келесі белгілі \(\displaystyle \angle ACB=124^{\circ}\) және \(\displaystyle \angle CAB=39^{\circ} {\small.}\) \(\displaystyle \angle DCB {\small}\) табыңыз. Жауабын градуспен беріңіз.
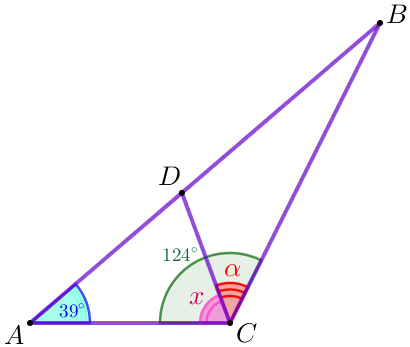 | Егер \(\displaystyle \angle DCB=\color{red} { \alpha} {\small,}\) \(\displaystyle \angle ACD=\color{Magenta}x{\small.}\) Сурет бойынша \(\displaystyle \color{red} { \alpha} = 124^ {\circ}- \color{Magenta}x{\small.}\) |
\(\displaystyle ACD\) – үшбұрыш – теңбүйірлі, онда \(\displaystyle \angle ADC=\angle ACD=\color{Magenta}x{\small.}\) Үшбұрыштағы бұрыштардың қосындысы \(\displaystyle 180 ^{\circ} {\small . } \) Онда \(\displaystyle \color{Magenta}{x}+ \color{Magenta}{x}+39^{\circ}= 180^\circ{\small , } \) \(\displaystyle 2 \cdot \color{Magenta}{x}= 180^\circ - 39^{\circ}{\small , } \) \(\displaystyle \color{Magenta}{x}= \frac{1}{2} \cdot 141^\circ=70{,}5^{\circ}{\small . } \) | 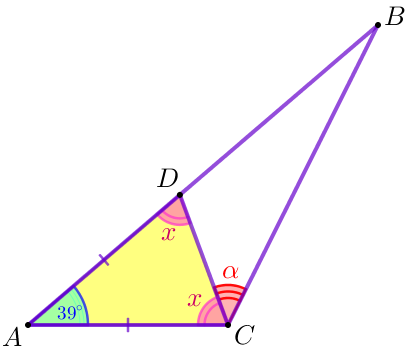 |
Аламыз
\(\displaystyle \color{red} { \alpha} = 124^{\circ}- \color{Magenta}x=124^{\circ}-70{,}5^{\circ}=53{,}5^{\circ}{\small.}\)
Осылайша, \(\displaystyle \angle DCB=53{,}5^{\circ}{\small.}\)
Жауап: \(\displaystyle 53{,}5 {\small.}\)