\(\displaystyle f(x)=(x-2)^2(x-4)+5\) кесіндісінде \(\displaystyle [1;\,3]{\small.}\) функциясының ең үлкен мәніне жеткен нүктені табыңыз.
Өрнекті жеңілдетейік \(\displaystyle (2x-4)(x-4)+(x-2)^2{\small.}\) Ол үшін жақшаларды ашып, ұқсас шарттарды беріңіз:
\(\displaystyle \begin{aligned}\color{green}{(2x-4)(x-4)}+\color{blue}{(x-2)^2}=\color{green}{(\color{red}{\underline{\color{green}{2x^2}}}-\color{red}{\underline{\underline{\color{green}{4x}}}}-\color{red}{\underline{\underline{\color{green}{8x}}}}+\color{red}{\underline{\underline{\underline{\color{green}{16}}}}})}+\color{blue}{(\color{red}{\underline{\color{blue}{x^2}}}-\color{red}{\underline{\underline{\color{blue}{4x}}}}+\color{red}{\underline{\underline{\underline{\color{blue}{4}}}}})}=\\[5px]=\color{red}{\underline{\color{black}{3x^2}}}-\color{red}{\underline{\underline{\color{black}{16x}}}}+\color{red}{\underline{\underline{\underline{\color{black}{20}}}}}{\small.}\end{aligned}\)
Осылайша, біз аламыз
\(\displaystyle f^{\prime}(x)=3x^2-16x+20{\small.}\)
2) \(\displaystyle f^{\prime}(x)=0{\small}\) болатын нүктелерді табайық.
\(\displaystyle f^{\prime}(x)=3x^2-16x+20{\small}\) болғандықтан, ол үшін теңдеуді шешу керек:
\(\displaystyle 3x^2-16x+20=0{\small.}\)
3) Туындының түбірлерін нақты түзуде белгілейміз, сонымен қатар оның пайда болған интервалдардағы белгілерін анықтаймыз.
- \(\displaystyle \color{green}{(-\infty;\,2)}\) және \(\displaystyle \textcolor{Purple}{\left(\frac{10}{3};\, +\infty\right)}\) аралықтарында \(\displaystyle f^{\prime}(x)>0{\small,}\)
- \(\displaystyle \textcolor{blue}{\left(2;\, \frac{10}{3}\right)}\) функциясы \(\displaystyle f^{\prime}(x)<0{\small}\) интервалында
Суреттегі туындының белгілерін белгілей отырып, біз аламыз:
4) Ережені пайдаланып \(\displaystyle f(x)=(x-2)^2(x-4)+5{\small}\) функцияның өсу және кему аралықтарын анықтайық.
Егер кез келген нүкте үшін \(\displaystyle x_0\in(a;\,b)\) туынды \(\displaystyle f'(x_0)\) бар болса және \(\displaystyle f'(x_0)>0{\small}\) болса, онда
\(\displaystyle f(x)\) функциясы бүкіл интервалда \(\displaystyle (a;\,b){\small}\) өсуде ( seаrrow )
Егер кез келген нүкте үшін \(\displaystyle x_0\in(a;\,b)\) туынды \(\displaystyle f'(x_0)\) бар болса және \(\displaystyle f'(x_0)<0{\small}\) болса, онда
\(\displaystyle f(x)\) функциясы бүкіл интервалда \(\displaystyle (a;\,b){\small}\) кемуде ( seаrrow )
\(\displaystyle f'(x){\small}\) Туындының белгілерін білу \(\displaystyle f(x){\small}\) өсу және кему аралықтарын анықтау
Схемалық \(\displaystyle f(x){\small}\) түрде көрсетіңіз
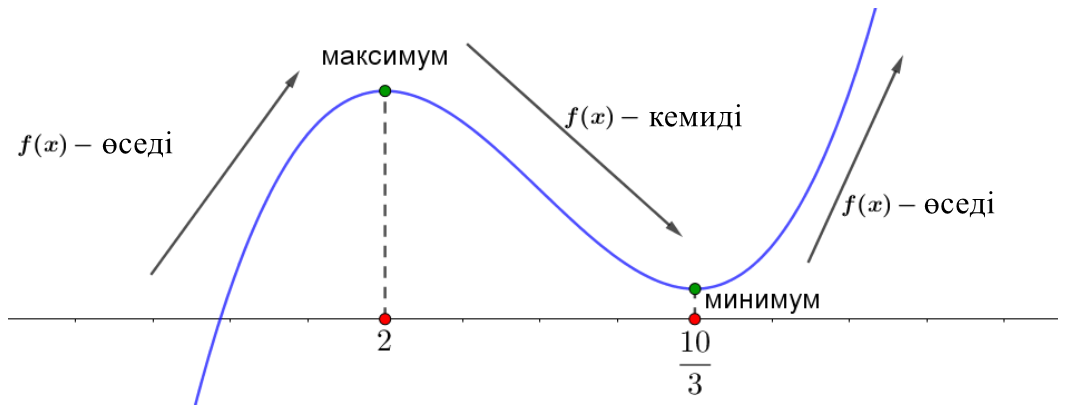
Демек, \(\displaystyle x=2\) – функцияның ең үлкен нүктесі \(\displaystyle f(x)=(x-2)^2(x-4)+5{\small.}\)
Ал нүкте \(\displaystyle x=\frac{10}{3}\) – ең кіші нүкте.
5) \(\displaystyle \left[1;\,3\right]\) аралық нүктелерінің қайсысында максималды мәнге жеткенін анықтайық.
Максималды нүкте \(\displaystyle x=2\) \(\displaystyle \left[1;\,3\right]{\small}\) интервалына түседі.
+ \(\displaystyle \color{green}{x=2}\) мәніне максималды нүктені \(\displaystyle \color{blue}{x=1}\) және интервалдың ұштарын \(\displaystyle \textcolor{Purple}{x=3}\) және \(\displaystyle f(x)=(x-2)^2(x-4)+5{\small}\) ауыстырыңыз:
- \(\displaystyle f(\color{green}{2})=(2-2)^2(2-4)+5=0^2\cdot(-2)+5=5{\small,}\)
- \(\displaystyle f(\color{blue}{1})=(1-2)^2(1-4)+5=(-1)^2\cdot(-3)+5=-3+5=2{\small,}\)
- \(\displaystyle f(\textcolor{Purple}{3})=(3-2)^2(3-4)+5=(1)^2\cdot(-1)+5=-1+5=4{\small.}\)
Ең үлкен мәнге \(\displaystyle \color{green}{x=2}{\small}\) нүктесінде жеткенін көреміз.
Жауабы: \(\displaystyle 2{\small.}\)
Суреттегі \(\displaystyle \left[1;\,3\right]{\small}\) аралықты белгілеңіз
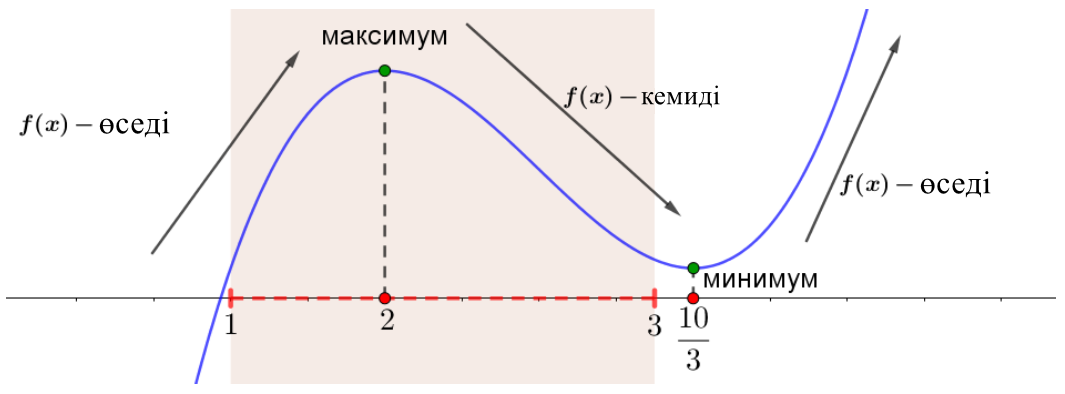
\(\displaystyle \left[1;\,3\right]\) кесіндісінде функция \(\displaystyle x=2{\small}\) нүктесіне дейін өсетінін, содан кейін кемитінін көруге болады.
Бұл ең үлкен мәнге дәл \(\displaystyle x=2{\small}\) нүктеде жеткенін білдіреді